LITL: “Magic” Ratio for Relationships

Posted on by
1

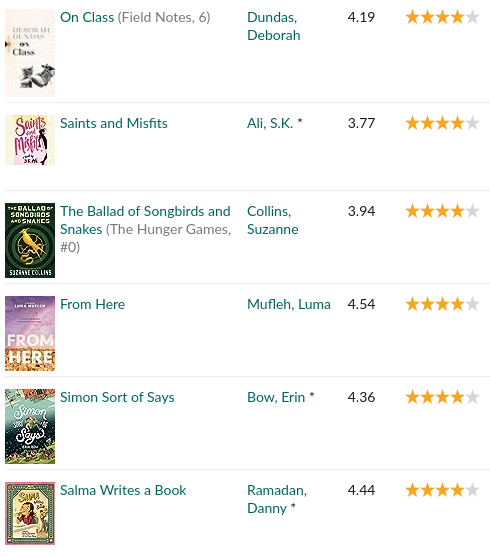
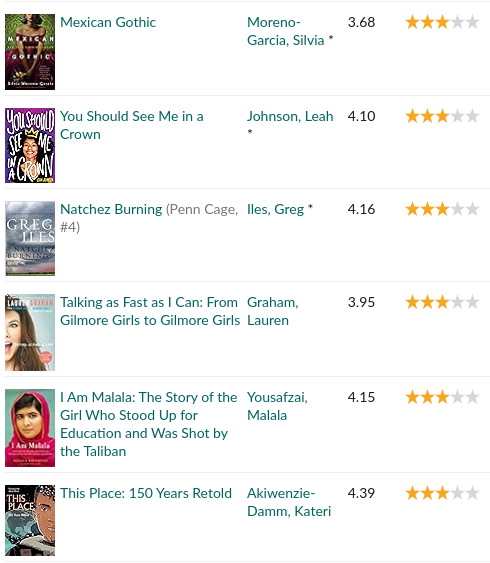
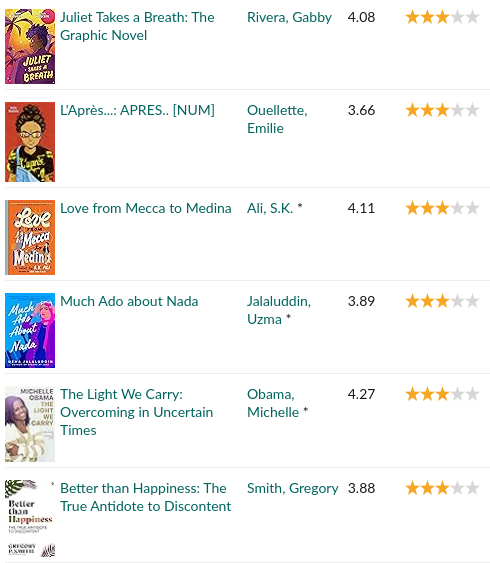
I just finished listening to the audiobook version of How Do We Know Ourselves? Curiosities and Marvels of the Human Mind by David Myers, a sort of pop psychology overview. My biggest takeaway was about Gottman’s research on the “magic” ratio for successful relationships; that the balance of positive interactions to negative ones during conflict should be at a 5:1 ratio.

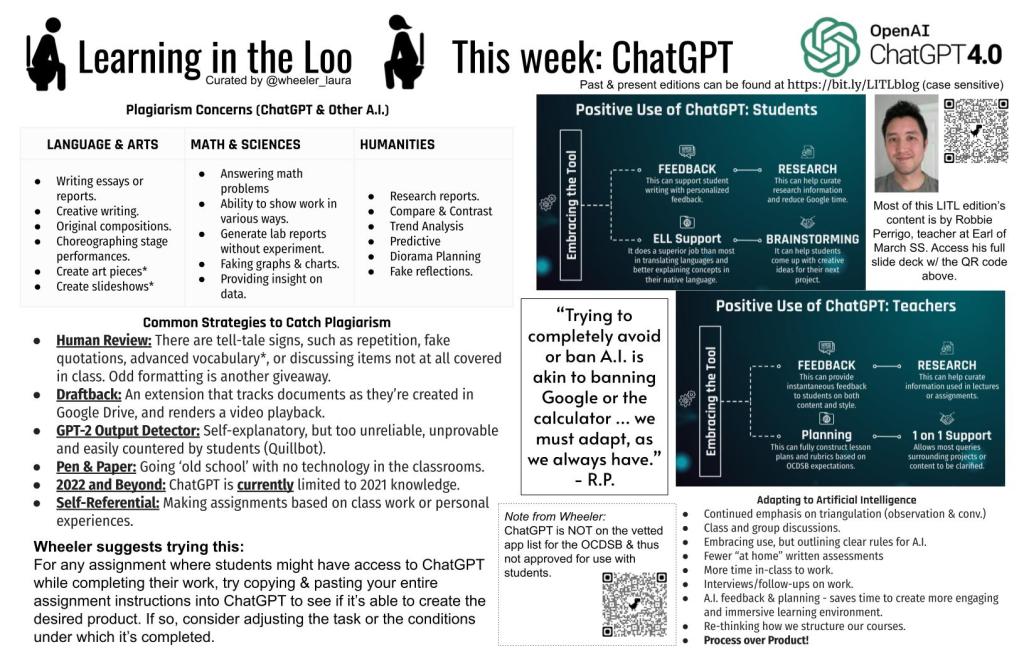
I started wondering about its applications in a school (or at least work) setting. So I did a little digging to look into it more & created this Learning In the Loo edition:

What Gottman’s research shows:

“The difference between happy and unhappy couples is the balance between positive and negative interactions during conflict. There is a very specific ratio that makes love last.

That “magic ratio” is 5 to 1. This means that for every negative interaction during conflict, a stable and happy marriage has five (or more) positive interactions.”
https://www.gottman.com/blog/the-magic-relationship-ratio-according-science/
“Outside of conflict discussions, successful couples had an even higher positive-to-negative ratio – 20:1.”

source: https://www.gottman.com/blog/the-magic-ratio-the-key-to-relationship-satisfaction/

But does it hold true outside of a romantic relationship?

“Research … looked at whether the Gottman ratio also applied to work relationships …The most important factor that differentiated the most and least successful teams was in fact the ratio of positive comments to negative comments the participants made to one another. The average ratio for the highest-performing teams was 5.6:1, positive to negative. The medium-performance teams averaged about 2:1, positive to negative. But the average for the low-performing teams was 1:3: These groups experienced almost three negative comments for each positive one.

The takeaway, for both leaders and peers, is simple: If you want to be part of a high-performing team, pay attention to how much praise and positivity you’re doling out. If your ratio is below 5:1, you may be damaging the effectiveness of your team.”

source: https://www.psychologytoday.com/ca/blog/curating-your-life/202206/the-gottman-ratio-happy-relationships-work

Consider: How might these ratios apply to the relationships we build with our students?


“What are some examples of interactions that could result in that negative emotional state?

Use of the Four Horsemen:
– Defensiveness,
– Criticism,
– Contempt,
– or Stonewalling
Lashing out at your partner when flooded instead of taking a time-out
Raising your voice during conflict
Neglecting to do something you told your partner you would do
Being invalidating
Rejecting bids for connection
Using a harsh start-up
Rejecting a repair attempt used by your partner
Not listening to your partner when they speak during conflict
Forgetting important milestones and events that are important to your partner”


“…here are some tips to have more positive interactions:

Share Appreciation
Show affection often
Be an active listener
Engage in acts of service
Show your partner you are thinking of them
Show validation
Have fun together
Respond positively to bids for connection
Practice empathy”

Source: https://www.gottman.com/blog/the-magic-ratio-the-key-to-relationship-satisfaction/

As always, all my past editions (including this one) of Learning in the Loo can be found here.

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

What I Read in 2023

Posted on by
Reply

A little roundup of what I read in 2023. And some general blog thoughts about my reading routines below. All of these graphics come from my stats on Goodreads.

I always struggle to pick a star rating for what I read. So take it with a grain of salt. The yellow stars are my rating & the average is from all Goodreads users:

I think those last two I just never bothered rating, rather than being 0 stars.

Favourites

I think if I had to pick favourites, it would be two:
No Fixed Address: a middle school book that was really a clever read about the topic of a boy and his mum that live in their van & how he navigates that with school & his social life. Great exploration of the issue of being unhoused from the POV of a tween.
and
What We Saw: a pretty difficult read as it deals with teen rape, but I thought it was a really interesting way to tackle the topic as it did so from the perspective of another girl who was at the party, and not the victim herself. Plus I thought it was a pretty realistic look at teen lives & social interactions.

What / Who do I read?

I read a mix of fiction (physical books) – both adult & YA/middle school, graphic novels (more one-off stories rather than manga series), and non-fiction (always in audiobook format). I read books folks recommend to me, ones I’ve seen recommended for high school libraries, ones that are in our school library that look good & interesting. I tried to read more muslim authors this year as I was also trying to build our collection of muslim authors in our library (mostly by me buying the book, reading it, then donating to our school library since there hasn’t been any budget allocated to us in 2 years). I think my reading list is fairly diverse, but if you spot any holes in what I read above, please let me know in the comments! Every year for a while now, I try to read all 5 books on the Canada Reads shortlist before the radio debates begin; that list comes out tomorrow!!

Where do I get my books?

Once upon a time I bought all my books as I wanted to have bookshelves full of every book I’ve ever read. Since our latest house move, I pared down. Those bookshelves are now in a guest bedroom where folks, unless staying over, don’t see them. So I gave away everything but our favourite / meaningful books. Our absolute faves line a shelf in the living room where folks can see them; hopefully it gives a little insight into who we are & what we like. The rest I donated to our school or other people.

So now I try to borrow books from our school library or the public library (I purchase an Ottawa library card annually to have access to their collection which is bigger than the library in my small village, especially audiobooks). Some I purchase when I want to read them now, but they aren’t available to borrow yet, or if I know I want to donate it to our school library so our students have access to it. I dropped my subscription to audible & just purchase from the app when I can’t get an audiobook from the public library (more rare than the number of free credits I got with the purchase plan).
I am subscribed to the free ALC (audio listening copies) program for educators & librarians from Libro.FM, which I love. Some ALCs & graphic novel ARCs I’ve gotten from NetGalley but haven’t done so lately – I should get back on there. But I don’t do fiction from there as I’d rather be off screen for the majority of my reading.

When do I read?

Most days I get some reading time in before bed. I try to make sure my last 30 minutes before sleep are off screens, so reading a physical book in bed is perfect! I have also started doing screen-free Saturdays so I tend to read a lot more on that day of the week. I listen to my audiobooks while driving, cooking, or doing other chores around the house. I don’t think I spend all that much time reading, but others suggest I must based on my annual book count.

What was your favourite book of 2023?
What book do you want to read in 2024?
What reading routines do you want to establish for the coming year?
Let me know in the comments!

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

2023 Review of My Year on Twitter

Posted on by
1

Each year I try to look back at my top tweets as a way of reflecting on what I’ve shared on Twitter. Twitter analytics is a great tool for this. It uses stats of views, replies and retweets to determine your top tweets. Some I include here because they got a lot of views, others got fewer views but had a high “engagement” rate. Here we go . . .

Each year I keep a thread of the books I’ve read pinned to the top of my profile & here was the first book of 2023; a pick from the free library at my mum’s community pool house when I visited her in Florida over winter break.

Doing my best to drum up an audience for my OLASC session:

This idea that my colleague, Ms. Lavallée, put into play caught a lot of eyes on Twitter:

Then the aforementioned library conference took place & that’s always likely to give me some top tweets for the month. A little tweet to try & get the hashtag flowing on the first morning of the conference:

Every year sketchnotes get lots of attention on Twitter:

This one got a quote tweet from keynote speaker Elamin Abdelmahmoud himself:

This post in support of nurses to start off the month of March:

And the wrap up to my 4 part Learning in the Loo series of Aleda Klassen’s amazing Thinking Classroom sketchnotes:

My lukewarm review of Lauren Graham’s audiobook (not the top tweet):

Thinking Classroom + sketchnote = popular tweet every time:

Some words of wisdom (sans sketchnote) from Peter Liljedahl:

When we chose to live at our cottage full time we knew it was a long commute. But we leave early in the morning to beat traffic and carpool together when we can. But the end of the school year saw some really terrible traffic days in part due to a school bus strike in Western Quebec:

After listening to a segment on the morning CBC show about cell phones in classrooms I tweeted this:

My final LITL poster of the year which also got featured on this podcast:

And again anything Thinking Classroom is popular – even just my reply to this tweet:

I won’t post this category of top tweets for every month, but last year (or the year before?) I started posting my weekly workout collage to Twitter (in addition to FB & IG where I’d been posting them):

July is a slower month for education content, so a more popular post was one of the books I read & keep in my annual “what I’ve read” thread. The books was This Place: 150 Years Retold:

Another Thinking Classroom related tweet:

Another book in the thread coming in top for the month. The Sleep-Deprived Teen: Why Our Teenagers Are So Tired, & How Parents and Schools Can Help Them Thrive by Lisa L. Lewis:

Then end of August our Breakfast Chat started back up for the new school year, asking about our best time we had over the summer:

And then back to school in September. Breakfast chat again:

My “top tweets” lately have far fewer impressions than ever before … are people just distancing themselves from what’s now called X (formerly known as Twitter?). My follower numbers haven’t significantly decreased that I can tell. Or just algorithm changes that don’t favour my tweets? Interesting as I have noticed myself feeling like the things I tweet aren’t getting the same interaction they used to. So “top” for the month, but not huge numbers, was:

This had some good engagement per view:

Teachers are hungry for more Thinking Classroom learning & materials so this tweet gained some serious traction (and still is seeing replies, reposts now at year’s end!).

Another BFC530 contribution:

While we’re at it, this is likely the last annual roundup of top tweets I’ll make as Twitter is kind of a ghost-town these days compared to the good old days when engagement was through the roof:

A couple last tweets that did the best in December to close us out:

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

LITL: ChatGPT

Posted on by
2

This week’s Learning in the Loo is all about ChatGPT for students & teachers. In our board, we cannot direct students to use ChatGPT because of age restrictions & privacy issues. But we all know many students are using it. It can also be a helpful tool for teachers too. I ran a Lunch N Learn for staff about ChatGPT earlier this week but many busy teachers couldn’t make it, so I decided to follow up with an edition of Learning In the Loo as well.

Huge shout-out to Robbie Perrigo, a colleague in my board, for giving me permission to use his slidedeck on the topic to create this edition.

As always, all my past editions (including this one) of Learning in the Loo can be found here.

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

Screen Free Saturdays

Posted on by
1

A few years ago now, I blogged about ways I was creating balance in my digital life:

  1. Setting 30 minute daily timers on social media apps that then lock the app,
  2. Turning off notifications for most apps other than text, phone & personal email,
  3. Keeping screens out of the bedroom, and
  4. No screens for the first & last 30 minutes of every morning & evening.

This February my curiosity was piqued about a book suggested in Vickie M Lanthier’s email newsletter; 24/6: The Power of Unplugging One Day a Week by Tiffany Shlain. I got the audiobook version (my preferred format for non-fiction) from the library & by the end of the book I was convinced I needed to add a screen-free day to my weeks. Since I work at school Mondays through Fridays, and at a horse farm all day Sundays … that left Saturdays for my screen-free days. So late March 2023 I had my first Screen-Free Saturday:

Here’s how I do my screen-free day: When I go to bed on Friday evening I put my phone into airplane mode. It stays that way until Sunday morning when I wake up. I make an exception to use my phone’s camera during my screen-free days, but I don’t edit the photos, look through them or post them. Take the photo & then phone away. I make an effort to not keep my phone on me, in my pocket, around the house when screen-free – I can go grab it when I want a photo.

Usually when I cook (or do other household chores) I listen to an audiobook to help the time pass faster. So I bought a little CD player and brought back out my old cases full of CDs from the 90s & 2000s. So on Saturdays I play CDs instead of listening to something from my phone. This past weekend, my partner was away, so I caught up on past editions of our local weekly newspaper, the LowDown, as I ate breakfast.

With the whole day ahead of me I often get some household chores done (that used to get postponed b/c I’d not have enough hours in my Saturday!). Sweep, laundry, change the sheets …

I’ll do tasks I don’t have time for during the week like meal prepping my breakfasts. This past weekend I spent 3 hours hand painting the lettering on the street signs for our private roads. I also finally got around to painting a design on the lamppost my husband made out front of our house this summer; the design has the same colours and outer shape as the barn quilt that hangs on our house.

Of course naps, with or without our cat Sly, feature on the agenda 🙂

During the week I workout to videos by fitness trainers from a video-exercise platform. So on screen-free Saturdays I try to pick a workout outdoors; go for a walk, a jog, or a paddle, etc. If the weather is really bad, I’ll use my spin bike, with the screen off, and read a book as I pedal.

In the evening, if there’s hockey my husband watches that on TV upstairs & I read downstairs. If there’s no hockey we’ll play scrabble or cards.

Until this past weekend, I mostly hadn’t been doing my Screen-Free Saturdays once school started because I was working on my illustrations for the next Thinking Classroom book. And boy did I miss screen-free time! Every screen-free Saturday is like a breath of fresh air in the week. No phone, no computer, no TV. More time for me and my partner, more time spent outside, more time spent caring for our home. We live full-time in our cottage, a move we made just before the pandemic hit. My Screen-Free Saturdays bring me back to when we didn’t yet live here full time; we had no cell service (still don’t) & no internet here. Simpler, more relaxing times. I’m bringing them back.

Are you taking any screen-free or disconnected breaks? Tell me about it in the comments!
I’ve even started to put my phone in airplane mode some evenings while we watch TV or read, just to give me a break from social media & text distractions that might come in.

Would you want to try a screen-free day? Maybe just a morning or afternoon?
What day of the week could you go screen-free?

– Laura Wheeler

How to Instantly Feel Better

Posted on by
1

I saw a version of this on Instagram the other day. I really liked the message & wanted to create a sketchnote version of it. But I couldn’t figure out who the original author was (there are many visuals of this out there) , so pls let me know if you know the original author.

How to instantly feel better:
Overthinking → Write
Anxious → Meditate
Tired → Nap
Sad → Exercise
Stressed → Go for a Walk
Angry → Listen to music
Lazy → Reduce screen time
Burnt out → Read

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

#LITL: Diffit for levelled reading resources

Posted on by
Reply

My goal this year was to get a new Learning In the Loo poster out once every week or two. It’s already been a month I think since I put my first one up 🤣

Earlier this year I read about a new (I think new?) site called Diffit that can create levelled reading resources based on topics or provided texts. In a school like mine that has both ESL & ELD students in many course sections, this can be a big time saver for teachers looking to find resources at the reading level of their students. And literacy is a big focus for our school board this year.

So I grabbed a few images to help explain what Diffit does & posted these in every teacher toilet stall in the building:

As always, all my past editions (including this one) of Learning in the Loo can be found here.

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

Library Year in Review 2022-23

Posted on by
1

In June of last school year I created my first “Library Year in Review” that outlines all the great happenings in our school library for the year. I got the idea from Cathy MacKechnie at Nepean High School who creates one for her library every year. Following her lead, I sent ours to all the teachers at our school, the parent-school council, our admin team (Ps & VPs), our superintendent, plus a few more folks. While I received some nice feedback on it from a few teachers, I was disappointed not to hear anything from our admin team or superintendent, especially given the great things that happened in our library despite being given 0$ in budget for the year! I will still absolutely do this again this school year and I thought it might be worth sharing here on the blog as well:

Hi folks,

Thank-you to all of you that brought students and classes to the library this year. A little summary of our year here in the library to share . . . 

We now have an amazing “Thinking Classroom” space with lots of whiteboards and a new projector set up in seminar B to make it a true classroom space (thank-you Brian & José for the installs). Classes came to participate in workshops on:

  • research skills
  • student vote
  • formal citations
  • speed dating for books
  • time management
  • sketchnoting
  • general introduction to our library space

These are the top 10 books checked out from our library this year. You’ll notice a trend; graphic novels & manga series are the biggest hits!

Since manga series can be costly to add to our collection, and with no library budget this year, we started an online wishlist that allows folks to purchase books for our library that have been requested by our students or determined to meet our goals of diversifying our collection to have more authors & characters that better reflect our student body. Our wishlist can be found here if you know of anyone who would be interested in donating to our library. We received a few donations already this year for which we are very thankful:

We implemented a new “free book” shelf outside the library doors where any student, staff or visiting community member can help themselves to free books to take home. These are books that are weeded from our collection because they haven’t been checked out in many years by our students, that we have more copies of than we require, or that are donated to the library but deemed not to fit the needs of our collection at this time. It’s been a popular attraction with books being taken home, and the shelf has been refreshed regularly all school year.

One of our big undertakings this year in partnership with Brittany Melia, the Teen Librarian at Greenboro – Ottawa Public Library, has been getting all of our students a public library card. We’ve handed out more than half of them so far with another batch still to come in. Students have told me this has been super helpful so far even just to access the members-only stronger wifi at their branch to download Mr. Lesser’s slide decks faster 😉 In September we’ll do the same for the incoming grade 9s & any new students. This means teachers can make use of the many online resources from OPL (like Curio, etc) as all students will now have the ability to login & access them.

We had our first author visit post-Covid! Local authors – one of whom is a Ridgemont parent – Alison Lister & ‘Nathan Burgoine spoke with our students about writing books for teens, healthy relationships and dealing with systemic biases and microaggressions on the daily. Students had great questions for these authors on some very important topics.

They also kindly donated many copies of their books as well as others from their publisher, Formac-Lorimer, to our library & students:

Finally, I’d like to call attention to a portion of a letter written by the OSSTF Library Subject Council (OCDSB Secondary Teacher-Librarians) to Michele Giroux, Director of Education, advocating on behalf of OCDSB Libraries with respect to the 2023-2027 Strategic Plan: 

“The Library Learning Commons can be a significant strategic player in addressing equity issues among students, because we are a central, welcoming, unstigmatized hub that all students use, regardless of economic or social factors.

However, many Library Learning Commons do not have adequate budgets or staffing to provide . . . a resource collection that can respond in a complete and up-to-date manner  to students’ needs, cultures, and identities.

Larger schools with larger budgets can provide expensive digital resources and help students develop skills for using them, whereas smaller and less affluent schools can not provide this access or this education.  . . . 

Furthermore, Library budgets should be protected and prioritized to ensure that students across the OCDSB are experiencing equitable access to resources.  There should be a per-student funding model, based on the Ministry allocation of Library funds that is designated directly for purchases within the Library Learning Commons at each school site.  A consistent funding model would allow for stable, long-range development in response to student needs at each school community level.”

Thanks for reading this far. Looking forward to new (& of course returning) students, new ideas & new partnerships next year with you all. Keep up the great work & see you in September.

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

Learning In The Loo: Google Classroom End of Year Cleanup

Posted on by
1

I wanted my last edition of the year to help teachers organise / clean up their Google Classroom. I went looking for what images were already out there on the interwebs & found this great infographic from Matt Miller of Ditch That Textbook :

I added some screenshots from my own Google Classroom & Calendar to help illustrate some of the items listed and included a QR code back to Matt’s original post which has more details about each of the tips on the list.

Are there any Google Classroom end of year tips you think are important that we missed here? Leave a comment below!

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)

Class Photo Project inspired by @KC_Adams_art

Posted on by
1

This past weekend I read the KC Adams art book Perception: A Photo Series. I was prompted to read it from a “further reading” list in the book Resurgence: Engaging With Indigenous Narratives and Cultural Expressions In and Beyond the Classroom from our teacher-librarian book club.

“Tired of reading negative and disparaging remarks directed at Indigenous people of Winnipeg in the press and social media, local artist KC Adams creates a body of work that documents another perspective.
This photo series called “Perception,” is an attempt to combat the stereotypes some of the public have of First Nation, Inuit and Metis people to illustrate, you can’t judge a book by its cover.
In the first photo, the models were asked to think about racist remarks they or their family have experienced such as the text written on the title of their photo. In the second photo, they were asked to think about a family member or a happy moment in their life and write their own self-identifying title
“I always felt that there were so many Indigenous People in Winnipeg who were leaders in their community and living normal or average lives. However their stories never made it into the newspapers or on social media. Then the scandal with Mayoral candidate Gord Steeve’s wife Lorrie Steeves broke in the media and I realized that racism is very much alive in Winnipeg. I decided to ask models to pose for me and offer them a chance to label themselves”. — KC Adams #perception — in Winnipeg, Manitoba.” Source

http://www.kcadams.net/art/photography/PERCEPTION.html

I was immediately struck by how this photo essay format could be emulated with students for any social justice issue being studied in a variety of classes. One would start by reading the book to the class – there are some great introductory essays that really set the tone of the project at the beginning of the book. Then look through all the photo sets, asking some discussion questions to really analyse the format of the photo sets, the words & terms chosen, the pattern used, the messaging, etc.

Then students would choose a social justice issue that they would like to explore (& related to the course) and start to plan who they might photograph for their models. I made a 1-pager that students could use to plan out each photo set:

Document available here

One would want to reach out to KC Adams before starting the project to ensure they are comfortable with students emulating the format in class. I could also see this format being used for more light-hearted comparisons or even objects with no social justice connotation (stereotypes about Canadian provinces in grade 9 geography maybe) but would absolutely want to check with the artist before taking it in that direction.

Would you try this with your class?
Either way I highly recommend this book – I borrowed it from the public library. Being mostly photos, it’s a quick read.

– Laura Wheeler (Teacher @ Ridgemont High School, OCDSB; Ottawa, ON)